POWER/INDUCTIVE
GENERAL CONSIDERATIONS
In theory, any (magnetic) material could be used for power conversion applications; however, there are specific material attributes that make some materials preferred over others. Frequency should be the first consideration in terms of performance. In order to get a general feel for a material’s potential as an efficient power material, one can simply start by considering the relative µ’ vs µ” response at the frequency of interest. The µ’ to µ” ratio at the operating frequency gives an idea of relative Q. A rule of thumb is for the ideal operating frequency to be Q > 50. Q is measured at low operating flux density (and at room temperature), so this is only a starting point.
The second consideration is the actual power loss (PL) vs. flux density (B) curves. Manufacturers supply specific power loss vs flux density curves (at constant temperature & sinusoidal conditions) for power rated materials. Specific power loss does not apply to any particular part or geometry but is measured on standard toroids as a material characteristic. On a component-by-component basis, power loss will be published as an absolute value, where Pabs= Pspecific * Volume. The volume could be the actual calculated geometric volume or (at times with more complex shapes) the effective magnetic volume (Ve = Ae * le). Geometric volume is more accurate, but with complex shapes variation of the cross-sectional area (Ae) creates hot spots where the actual cross-sectional area is at the minimum value (Amin) over the entire magnetic path and localized loss will be greater.
The most optimal materials will be those that demonstrate lowest power loss at the operating frequency, temperature, and maximized operating flux density. Other material attributes to consider are µ’ vs temperature (µT), power loss vs temperature (PL vs T), amplitude permeability vs flux density (µA or µ vs B), maximum flux density vs temperature (B vs T), and when the component is gapped (for applications where a DC bias consideration is required).
At relatively low frequency (<50 kHz), power capacity is mainly saturation flux density constrained. At higher frequency it is the losses over frequency that are the constraints. As the operating frequency increases, the maximum material flux density (B max) becomes less important. At low operating frequencies, there will be non-ferrite materials that have higher Bmax capabilities which will provide more efficient power conversion (alloys, amorphous and nanocrystalline materials). Currently, ferrite is the most efficient and cost-effective choice for higher frequency power conversion readily available.
It is always desirable to have flat performance over temperature: the µT and PL vs T curves give an idea of a material’s performance over temperature. Typically, most power materials have performance ratings characterized at 100°C, or the “sweet spot,” where power loss is at a minimum.
Fair-Rite Materials for Power Conversion
| material type | µ’ | Bmax (mT) | Tc (°C) | sweet spot (°C) | typical PL (mW/cc). | at MHz | at °C | at B (mT) | Frequency Range | B * f ref | B*f/PL |
| 77 | 2000 | 500 | > 200 | 80 | 130 | 0.025 | 100 | 100 | < 100kHz | 2.5 | 0.019 |
| 78 | 2300 | 480 | > 200 | 80 | 50 | 0.1 | 100 | 100 | 25 – 500 kHz | 10 | 0.200 |
| 95 | 3000 | 500 | > 200 | 60 * | 180 | 0.2 | 25 | 100 | 25 – 400 kHz | 20 | 0.111 |
| 79 | 1400 | 480 | > 200 | 70 | 100 | 0.5 | 100 | 50 | 300 – 1000 kHz | 25 | 0.250 |
| 80 | 600 | 480 | > 200 | 25 | 130 | 2 | 25 | 30 | 1 – 5 MHz | 60 | 0.462 |
| 61 ** | 125 | 235 | > 300 | 25 | 300 | 5 | 25 | 12 | 2-15 MHz | 60 | 0.200 |
| 67 ** | 40 | 240 | > 400 | 50 | 350 | 10 | 25 | 10 | 2-25 MHz | 100 | 0.286 |
The 95 material (*) has been developed for minimal PL variation over temperature. Note that the type 61 and 67 materials (**) are perminvar types. These materials, while providing very desirable low loss and relatively flat temperature response, are susceptible to ambient and operating conditions. Aside from external influences (mechanical stresses and external magnetic fields), operation must be limited to where the power loss won’t exceed 1W/cc. Above this point these materials can suffer from thermal runaway and the performance will be permanently compromised.
As a manufacturer, Fair-Rite publishes all power loss information performed under frequency-specific sinusoidal conditions. In many applications, the existing currents and voltages are not sinusoidal, so other estimations and algorithms (MSE, iGSE, Herbert plot, etc.) should be considered.

Operating at higher frequencies allows lower operating flux density for the same power levels and/or smaller component volume and/or mass. A general rule of thumb is to limit operation for 500mW/cc or less to ensure low component temperature rise (dT< 50°C). 300mW/cc to 1000mW/cc can also be used as operational limits depending on the cooling capacity of the system. The performance factor is a guide for: frequency * flux density vs frequency at temperature and specific power loss.
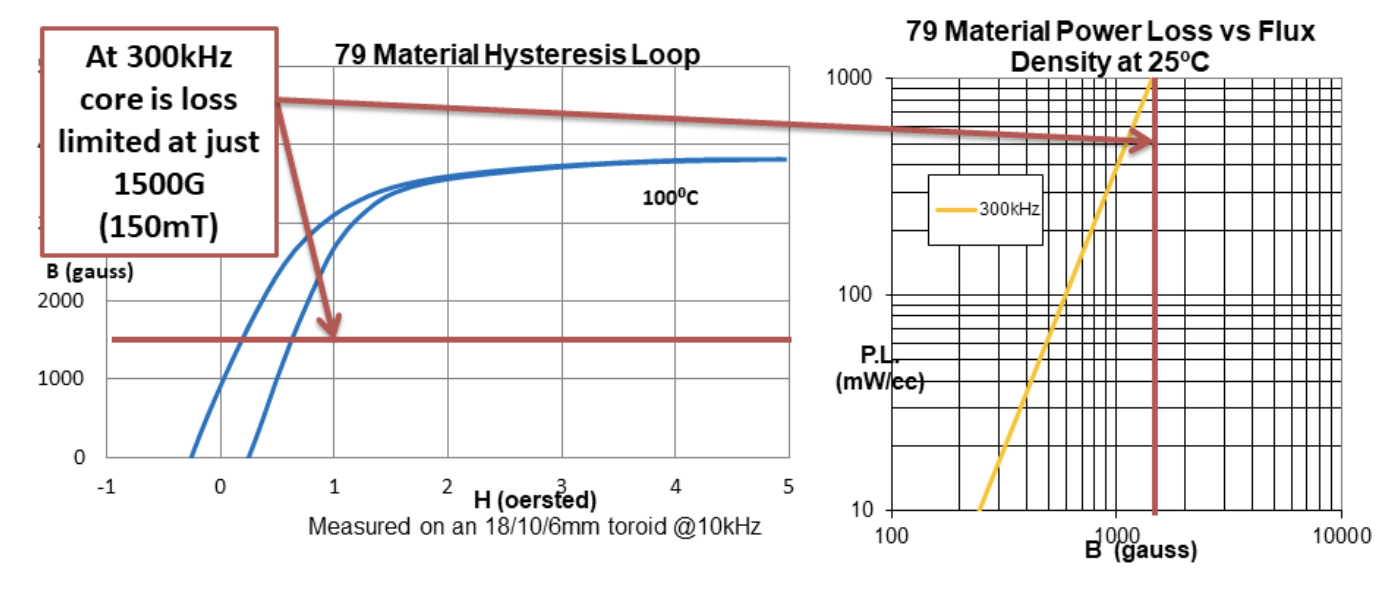
Hysteresis Loops can be a fair guide for a material’s ability to deliver maximal flux density (B). This is especially true at low frequency, but at frequencies above 100 kHz they can’t be relied on. The reason for this is that the loop changes as frequency increases. Temperature also changes this. A general rule of thumb is to limit ΔB excursions to no more than 1/2 the published Bmax (measured at 1 or 10kHz). As frequencies increase, operating ΔB should be further decreased to limit core loss. This also ensures reasonably low waveform distortion. There are three primary modes for the loss of a ferrite material; residual loss is inherent to the material and a constant, hysteresis losses are amplitude (B) dependent, and eddy current loss is a function of the material’s complex permeability and permittivity and change with frequency. Other losses realized are heavily influenced by the winding of the core.
Ferrite materials lend themselves well to a wide variety of power applications. Low losses and high operating frequencies put them in a league of their own for high efficiency, high power density designs. Their successful implementation hinges upon having as complete an understanding as possible of the operational parameters and being able to leverage this knowledge to select an optimal material and geometry.